A tangent line is just a line that touches the graph once. Here is a graph called f with a point labeled x and we calculate the slope of the tangent line at the point x with the difference quotient. The length from the origin to x is called "x." The coordinates at that point x (that is touching the tangent line) is (x,f(x)). Another point to the right of x is called "h(delta x or change in x)". So this new point has the coordinates of (x+h,f(x+h)). A secant line is a line that goes through two points. To find the slope of the secant line you need to use the point slope formula, which is y2-y1/x2-x1. Then, you just plug in the x and y values and you get f(x+h)-f(x)/ x+h-x. The x's will cancel in the denominator and you are left with f of x plus h minus f of x divided by h that's the difference quotient! So the difference quotient represents the slope of the secant line. The smaller the change in x, the more the secant line resembles the tangent line. To make the change in delta x smaller we use a limit in the secant slope. So we take the limit as h(delta x) approaches 0 because we want our delta x to be as small as possible for the secant line to resemble the tangent line. We can take the limit as h approaches 0 because approaches means getting close, but never getting there and that's exactly what we need (to get close to zero but not zero because we can't have 0 in our denominator). The difference quotient is the definition of the derivative.
Tuesday, June 3, 2014
Monday, May 12, 2014
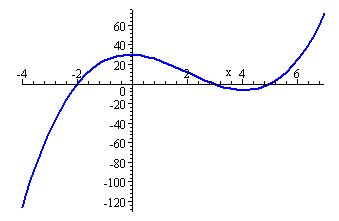
BQ#6 Unit U Concept 4:Continuous functions and types of discontinuities and limits numerically and graphically
1. A continuous function is predictable. It has no breaks, no holes, and no jumps. A continuous function can be drawn with a single, unbroken pencil stroke. Also, a continuous function is when the value is equal to the limit.
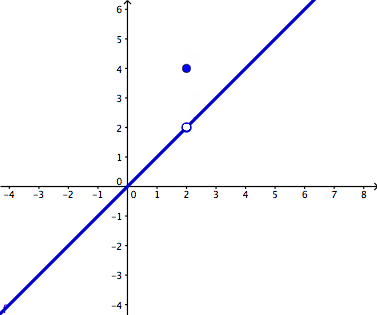
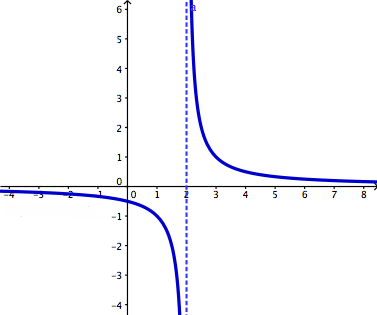
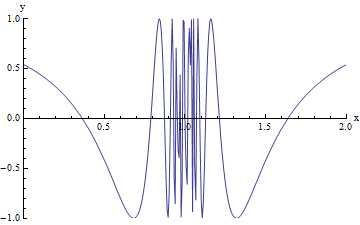
A discontinuous function is the opposite of a continuous function. It may contain breaks, holes, jumps. Also, the value does not equal the limit. There are two families of discontinuities: removable and non-removable discontinuities. In the removable family there is only point discontinuity. In removable discontinuities the limit DOES EXIST. In the other family, non-removable discontinuities the limit DOES NOT EXIST. The non-removable discontinuities family consists of jump discontinuity, oscillating behavior, and infinite discontinuity. In the jump discontinuities one hole has to be close and one open or both open or it will not be a function if both of the holes are closed. The reason the limit does not exist in jump discontinuities is because it approaches different values from the left and right. In oscillating behavior the graph is very wiggly and the limit does not exist because it doesn't approach any single value. Infinite discontinuities are caused by vertical asymptotes and the limit does not exist because of unbounded behavior (can't touch asymptotes) and because it approaches different values from the left and right.
2. A limit is the intended height of a function. The height is just the y-value of the function. Different x-values have different heights. A limit exists as long as you reach the same height from both the left and right. In order for a limit to exist, both the right hand limit and the left hand limit must be the same. If a graph does not break at a given x-value, a limit exists there. A limit can still exist if the destination is a hole in the graph. A graph can have an infinite amount of limits. Limits do not exist at specific points in the three non-removable discontinuities-jump, infinite, and oscillating. The limit does not exist at jump discontinuities because it approaches different values from the left and right. The limit does exist at infinite discontinuities because of unbounded behavior (can't touch asymptotes) and it approaches different values from the left and right. The limit does exist at oscillating behavior because it does not approach any single value because infinity is not anything you can get to. The difference between a limit and a value is the actuality. A limit is the intended height, while a value is the actual height.
3.We evaluate limits numerically by typing in the given function into out y= screen in our graphing calculator. Then, pressing the graph button and then hitting the trace button and we put in the number that we given in the table and repeat for all the others. On the left side of the table the number should increase from out to inner and on the right side of the table the number should increase out to inner. Then, you write out the number that is the middle or what the numbers are approaching and that number will become the limit. After, that you just write it as limit notation. We evaluate limits graphically by putting your finger on a spot to the left and to the right of where you want to evaluate the limit. If your fingers meet up then that y-value will be you limit. If your fingers do not meet up, the limit does not exist. The possible reasons for why the limit does not exist is because different values from the left and right, unbounded behavior, and oscillating behavior.We can evaluate limits algebraically by using three different methods. The first method, is substitution which is the easiest way to go about and we should always try to see if we get a numerical value, #/0(undefined= LIMIT DNE because unbounded behavior),and 0/#(limit is zero). If we ever get 0/0 when using the substitution method that means it is indeterminate, which means not yet determined and we must use another method. The substitution method means you take the number limit is approaching and plug it anytime you see "x". The second method, is factoring we use this method when the substitution method gives us 0/0 and the numerator or denominator are factorable. When using the factoring method we factor the numerator and denominator and cancel common terms to remove the zero(hole) in the denominator and then we use direct substitution method to get the limit. The last method is the conjugate method, we use this when the substitution method gives us 0/0 and the numerator or the demoniator are not factorable. We evaluate the limit using the conjugate method by first multiplying the top and bottom by the conjugate of the numerator or denominator(we use the conjugate of the numerator/denominator depending wherever the radical is). Then we simplify by foiling(you don't multiply the non-conjugate part because you want things to cancel) and then things will cancel and then we use the substitution method.
 | |||||||||
| http://www.intmath.com/differentiation/1-limits-and-differentiation.php |
 | |
| http://www.wyzant.com/resources/lessons/math/calculus/limits/continuity |
 |
| http://www.tutorvista.com/content/math/discontinuity/ |
 |
| http://www.wyzant.com/resources/lessons/math/calculus/limits/continuity |
 |
| http://www.cwladis.com/math301/limitsgraphically.php |
3.We evaluate limits numerically by typing in the given function into out y= screen in our graphing calculator. Then, pressing the graph button and then hitting the trace button and we put in the number that we given in the table and repeat for all the others. On the left side of the table the number should increase from out to inner and on the right side of the table the number should increase out to inner. Then, you write out the number that is the middle or what the numbers are approaching and that number will become the limit. After, that you just write it as limit notation. We evaluate limits graphically by putting your finger on a spot to the left and to the right of where you want to evaluate the limit. If your fingers meet up then that y-value will be you limit. If your fingers do not meet up, the limit does not exist. The possible reasons for why the limit does not exist is because different values from the left and right, unbounded behavior, and oscillating behavior.We can evaluate limits algebraically by using three different methods. The first method, is substitution which is the easiest way to go about and we should always try to see if we get a numerical value, #/0(undefined= LIMIT DNE because unbounded behavior),and 0/#(limit is zero). If we ever get 0/0 when using the substitution method that means it is indeterminate, which means not yet determined and we must use another method. The substitution method means you take the number limit is approaching and plug it anytime you see "x". The second method, is factoring we use this method when the substitution method gives us 0/0 and the numerator or denominator are factorable. When using the factoring method we factor the numerator and denominator and cancel common terms to remove the zero(hole) in the denominator and then we use direct substitution method to get the limit. The last method is the conjugate method, we use this when the substitution method gives us 0/0 and the numerator or the demoniator are not factorable. We evaluate the limit using the conjugate method by first multiplying the top and bottom by the conjugate of the numerator or denominator(we use the conjugate of the numerator/denominator depending wherever the radical is). Then we simplify by foiling(you don't multiply the non-conjugate part because you want things to cancel) and then things will cancel and then we use the substitution method.
Friday, April 18, 2014
BQ#4 Unit T Concept 1-3: Graphing all six trig functions
A normal tangent is uphill and a normal cotangent is downhill because of the placement of the asymptotes. For tan it goes uphill because we have asymptotes where cos equals zero(since tan's trig identity is sin/cos and we need to have zero in the denominator to get undefined=asymptotes) and remembering that we need to be in between the asymptotes but not touch them and tan needing to be positive in the first quadrant (because the sin and cos graphs being positive in quadrant one and a positive divided by a positive is positive=tan ) and tan needing to be negative in quadrant two, uphill is the only way you can fit the graph in between the asymptotes. For cot it goes downhill because we have asymptotes when sin equals zero(cot=cos/sin). Cot needs to be positive in the first quadrant(sin and cos are both positive) and negative in quadrant two(sin is postive and cos is negative; positive divided by a negative=negative) and downhill is the only way we can fit the graph in between the asymptotes and it be positive and negative in the second quadrant. To bring it all together the placement of the asymptotes makes a normal tangent uphill and a cotangent downhill.
BQ#3 Unit T Concept 1: Graphing all six trig functions
A.) Once, we draw the sin and cos graphs(sin graph starting and ending at 0 and cos graph starting and ending in its amplitude), we notice that the sin and cos graph are both positive in quadrant one, so the tangent graph is positive because if tan is sin/cos and sin and cos are positive a positive divided by a positive is positive.In quadrant two, the sin graph is positive and the cos graph is negative, so the tan graph is negative because a positive divided by a negative is negative. In quadrant three, the both the sin and cos graph are negative therefore the tan graph is positive because a negative divided by a negative is a positive. In quadrant four, sin is negative and cos is positive, so the tan graph is going to be negative. Tangent has an asymptote whenever cos equals zero. The asymptotes are determined with sin and cos. Asymptotes happen when we get undefined. We get undefined when we divide by zero or when cos is zero because tan is sin/cos.
B.)We know cot is cos/sin by our trig identities. Looking at quadrant one, we noticed both the sin and cos graphs are positive, so the cot graph will be positive. Cotangent is going to have different locations for asymptotes as opposed to tangent.Cotangent is going to have asymptotes whenever sin equals one. In quadrant two, sin is positive, but cos is negative so the cot graph will be negative. In quadrant three, both sin and cos are negative so cotangent is going to be positive. In quadrant four, cos is positive and sin is negative so cotangent will be negative.The asymptotes are determined with sin and cos.Asymptotes happen when we get undefined. We get undefined when we divide by zero or when sin is zero because cot is cos/sin.
C.)Secant is the reciprocal of cosine. In quadrant one, secant is positive and it goes very high up because if you take the reciprocal of a small fraction you will get a really big number. Sec is going to have asymptotes whenever cos equals zero.In quadrant two, cos is negative so sec is going to be negative because it needs to reflect off from the cos graph. In quadrant three, cos is negative, so sec is negative. In quadrant four, cos is positive so sec is positive.
D.)When sin equals zero there is going to be asymptotes. In quadrant one and two, sin is positive so csc is going to be positive or above the x-axis because it reflects off the sin graph.In quadrant three and four, sin is negative so csc will be negative or below the x-axis. The location of the asymptote shapes our graph. We have asymptotes when we get undefined and if we look at csc trig identity it is is 1/sin so sin has to be zero in order for us to get undefined which means asymptotes.
B.)We know cot is cos/sin by our trig identities. Looking at quadrant one, we noticed both the sin and cos graphs are positive, so the cot graph will be positive. Cotangent is going to have different locations for asymptotes as opposed to tangent.Cotangent is going to have asymptotes whenever sin equals one. In quadrant two, sin is positive, but cos is negative so the cot graph will be negative. In quadrant three, both sin and cos are negative so cotangent is going to be positive. In quadrant four, cos is positive and sin is negative so cotangent will be negative.The asymptotes are determined with sin and cos.Asymptotes happen when we get undefined. We get undefined when we divide by zero or when sin is zero because cot is cos/sin.
C.)Secant is the reciprocal of cosine. In quadrant one, secant is positive and it goes very high up because if you take the reciprocal of a small fraction you will get a really big number. Sec is going to have asymptotes whenever cos equals zero.In quadrant two, cos is negative so sec is going to be negative because it needs to reflect off from the cos graph. In quadrant three, cos is negative, so sec is negative. In quadrant four, cos is positive so sec is positive.
D.)When sin equals zero there is going to be asymptotes. In quadrant one and two, sin is positive so csc is going to be positive or above the x-axis because it reflects off the sin graph.In quadrant three and four, sin is negative so csc will be negative or below the x-axis. The location of the asymptote shapes our graph. We have asymptotes when we get undefined and if we look at csc trig identity it is is 1/sin so sin has to be zero in order for us to get undefined which means asymptotes.
Thursday, April 17, 2014
BQ#5 Unit T Concept 1-3: Graphing all six trig functions
To begin with, the Unit Circle ratios for sin is y/r, cos is x/r, csc is r/y( because it is the reciprocal of sine), sec is r/x(because it is the reciprocal of cos), tan is y/x, and cot is x/y (because it is the reciprocal of tan). With that being said, we have amplitudes when we are dividing by zero, which will give us defined because we can't divide by zero. Sin and cos never have asymptotes because if we look at their Unit Circle ratios, r is always in the denominator. As we know when we are dealing with the Unit Circle, r is always 1. Therefore, we never divide by zero, which we do not have amplitudes because it is never undefined because we do not divide by zero.
Wednesday, April 16, 2014
BQ#2: Unit T Concept Intro: Graphing all six trig functions
Trig graphs relate to the Unit Circle because if we took the Unit Circle and unwrapped it, it will become a straight line and the four quadrants will become hash marks.
A.)So, for sine and cosine the period is 2 pi because sine's pattern is ++-- and cosine's pattern is +--+(based on All Students Take Calculus) and looking at these patterns it will take the whole rotation of the Unit Circle or all of the four quadrants for the pattern to begin to repeat itself. If we look at the Unit Circle half way at 180 degrees it is pi and at 360 degrees (which is all the way around) it will be 2pi and since it takes sine's and cosine's pattern to begin repeating itself after four quadrants, which is at 360 degrees and 360 degrees is 2pi. One time through their cycle is called a period and therefore sine and cosine have periods of 2pi. On the other hand, tangent and cotangent have periods of pi(180 degrees) because tan's and cot's pattern is +-+- and as you can see the pattern is repeated twice. So, after two quadrants(180 degrees) the period starts to repeat itself therefore it is pi, instead of 2pi.
B.)Knowing the fact that sin and cos have to be in between 1 and -1 and that the Unit Circle can extend (0,1 at 90 degrees), (0,-1 at 270 degrees), (1,0 at 0 and 360 degrees), and (-1,0 at 180 degrees) we can state that sine and cosine have amplitudes of one. Also, sin and cos both have r=1 as a denominator.
A.)So, for sine and cosine the period is 2 pi because sine's pattern is ++-- and cosine's pattern is +--+(based on All Students Take Calculus) and looking at these patterns it will take the whole rotation of the Unit Circle or all of the four quadrants for the pattern to begin to repeat itself. If we look at the Unit Circle half way at 180 degrees it is pi and at 360 degrees (which is all the way around) it will be 2pi and since it takes sine's and cosine's pattern to begin repeating itself after four quadrants, which is at 360 degrees and 360 degrees is 2pi. One time through their cycle is called a period and therefore sine and cosine have periods of 2pi. On the other hand, tangent and cotangent have periods of pi(180 degrees) because tan's and cot's pattern is +-+- and as you can see the pattern is repeated twice. So, after two quadrants(180 degrees) the period starts to repeat itself therefore it is pi, instead of 2pi.
B.)Knowing the fact that sin and cos have to be in between 1 and -1 and that the Unit Circle can extend (0,1 at 90 degrees), (0,-1 at 270 degrees), (1,0 at 0 and 360 degrees), and (-1,0 at 180 degrees) we can state that sine and cosine have amplitudes of one. Also, sin and cos both have r=1 as a denominator.
Wednesday, April 2, 2014
Reflection#1: Unit Q: Verifying Trig Identities
1.) To verify trig functions it means to prove that the equation on the right is true by showing that the left side equals to the right. In other words, taking an equation and simplifying it until it is exactly the same as the right side. Verifying trig functions can be challenging because there is no set or "correct" method to verify these trig function and all it comes down is to a pick and guess situation and if it takes you to a more difficult path, then you can reverse and try another method.
2.) The number one tip I could give is listen to Mrs. Kirch when she says that you need to study in order to remember all the trig ratios, identities, and Pythagorean identities. The most helpful trick I can give is if you are stuck and you can get things to be converted into sin and cos then you should take that route. Another, tip I can give is to substitute the variables into something that is different from all the sin, pluses, minuses,etc. because a + next to a tan can be confusing.
3.)When verifying trig functions the first thing that I think is can I take out a GCF. If I can then you might want to do that first because perhaps things start cancelling out right there and then. The next step I take is to see if I can substitute an identity. It can be one of the ratio identities, reciprocal identities, or Pythagorean identities. Then, I look if the denominator is a binomial and if it is I can multiply by the conjugate to the numerator and denominator. Another thing you can perform is to combine fractions with binomial denominator or separate fraction, but only when the denominator is a monomial. In addition, you can factor and if all fails you can resort to changing everything to sin and cos.
2.) The number one tip I could give is listen to Mrs. Kirch when she says that you need to study in order to remember all the trig ratios, identities, and Pythagorean identities. The most helpful trick I can give is if you are stuck and you can get things to be converted into sin and cos then you should take that route. Another, tip I can give is to substitute the variables into something that is different from all the sin, pluses, minuses,etc. because a + next to a tan can be confusing.
3.)When verifying trig functions the first thing that I think is can I take out a GCF. If I can then you might want to do that first because perhaps things start cancelling out right there and then. The next step I take is to see if I can substitute an identity. It can be one of the ratio identities, reciprocal identities, or Pythagorean identities. Then, I look if the denominator is a binomial and if it is I can multiply by the conjugate to the numerator and denominator. Another thing you can perform is to combine fractions with binomial denominator or separate fraction, but only when the denominator is a monomial. In addition, you can factor and if all fails you can resort to changing everything to sin and cos.
Tuesday, March 25, 2014
SP#7: Unit Q Concept 1: Using fundamental identities to simplify and verify expressions
Please see my SP7, made in collaboration with Chelsea Amezcua, by visiting their blog here. Also be sure to check out the other awesome posts on their blog.
Tuesday, March 18, 2014
I/D#3: Unit Q Cocept 1: Using fundamental identities to simplify and verify expressions(simple,one or two step identities)
INQUIRY ACTIVITY SUMMARY
1.)
a. An identity is a proven formula and fact that are always true. The Pythagorean Theorem is an identity because it is a proven formula that is true.
b. The Pythagorean theorem is x^2+y^2=r^2.
c. If you wanted x^2+y^2=r^2 to equal 1 you will need to divide r^2 to the other side and you are left with x^2/r^2 +y^2/r^2=1. However, we can rewrite that and say (x/r)^2+(y/r)^2=1 because of the distributive property of a power.
d. The ratio for cosine on the unit circle is x/r.
e. The ratio for sine on the unit circle is y/r.
f. We can plug in cosine for x/r in the Pythagorean theorem(x/r)^2+(y/r)^2=1 and sin for y/r. We can conclude that the Pythagorean theorem is cos^2theta+sin^2theta=1.
g. Sin^2theta+cos^2theta=1 is referred to as a Pythagorean identity because we can plug in cosine for x/r in the Pythagorean theorem (x/r)^2+(y/r)^2=1 and sin for y/r and it is still the Pythagorean theorem but in words because the trig function for cos was x/r and sin's trig function was y/r. Therefore, it is the same as (x/r)^2+(y/r)^2=1 because we know the trig function for cos was x/r and sin's trig function was y/r.
2.)a.
b.
INQUIRY ACTIVITY REFLECTION
1. The connections that I see between Units N, O, P, and Q so far are the unit circle provided us with the ordered pairs of trig function and then we wan use those trig functions to solve for missing angles and sides of special right triangles and non-right triangles and now we can use the unit circle trig functions and ordered pairs to rewrite the Pythagorean theorem. Perhaps, we can use those trig functions and the Pythagorean theorem to now find missing angles and sides of special right triangles.
2.If I had to describe trigonometry in THREE words, they would be algebra geometry combined.
Sunday, March 16, 2014
WPP#13&14: Unit P Concept 6-7: Applications with Law of Sines and Cosines
This WPP#13-14 was made in collaboration with Chelsea Amezcua. Please visit the other posts on their blog by going here.
1.)Amarie Alize and Steven met today at a mutual friends' Super Bowl party. Before the game started the host decided to have a football game among all the friends. However, no one could find the football. Everyone was trying to look for the football(which was to the north of them) and at the same time Amarie Alize and Steven(who are 25 yards apart) found it. Steven saw the ball's path to be N28E from a north-south line through where he is standing and Amarie Alize saw the ball's path to be N39W from a north-south line through where she is standing. What is the distance between Amarie and the football?
2.)Steven and Amarie were playing football and they both run to catch the ball. Steven runs 12 yds at a bearing on 56 degrees and Amarie runs 14 yds at a bearing of 315 degrees. How far apart were they before they started running?
1.)Amarie Alize and Steven met today at a mutual friends' Super Bowl party. Before the game started the host decided to have a football game among all the friends. However, no one could find the football. Everyone was trying to look for the football(which was to the north of them) and at the same time Amarie Alize and Steven(who are 25 yards apart) found it. Steven saw the ball's path to be N28E from a north-south line through where he is standing and Amarie Alize saw the ball's path to be N39W from a north-south line through where she is standing. What is the distance between Amarie and the football?
2.)Steven and Amarie were playing football and they both run to catch the ball. Steven runs 12 yds at a bearing on 56 degrees and Amarie runs 14 yds at a bearing of 315 degrees. How far apart were they before they started running?
BQ#1: Unit P Concept 1-2: Law of Sines SSA and Area of an oblique triangle
| www.ck12.org/book/CK-12-Trigonometry-Concepts/r2/section/5.4/ |
References:http://www.ck12.org/book/CK-12-Trigonometry-Concepts/r2/section/5.4/
Wednesday, March 5, 2014
WPP#12: Unit O Concept 10: Solving angles of elevation and depression word problems
1. It is Fourth of July and Amarie Alize is looking up at the fireworks from
ground level. She estimates the angle of elevation from where she is now
to the fireworks up in the sky to be 23. She was watching the fireworks
that her neighbors were exploding illegally. She knows the distance
from where she stands to her neighbor house(where the fireworks are
coming from) is 100 feet. How far up are the fireworks exploding?(Round to the tenth place)
2. Amarie Alize took some ski lessons during the winter, but instead of going to the rookie slope she went to the advanced slopes. She is just about to ski down a steep mountain. She estimates the angle of depression from where she is now to the bottom of the mountain to be 30. She read a sign that said "500 ft above ground level." How long is the path that she will ski?
 |
| http://www.bing.com/images/search?q=fireworks&go=&qs=ds&form=QBIR#view=detail&id=90331943C86B19A045E9F3CB827184526A6E3CCD&selectedIndex=12 |
 | ||||
| http://www.bing.com/images/search?q=steep+mountain&qs=IM&form=QBIR&pq=steep+mountain&sc=8-14&sp=1&sk=#view=detail&id=2973C31EE05CF2709444D44E1B40C67FFD5D2CEE&selectedIndex=1 |
Monday, March 3, 2014
I/D#2: Unit O Concept7-8: Deriving the patterns for the 45-45-90 and 30-60-90 special right triangles
INQUIRY ACTIVITY SUMMARY
#1-3 are performed in the video
We cut the first triangle diagonal because it will form a triangle no like if you cut it straight it will give you a rectangle. We cut the second triangle straight because it will form a triangle and not another shape when cut horizontal or diagonal.
INQUIRY ACTIVITY REFLECTION
1. Something I never noticed before about special right triangles is that we can get the patterns for special right triangles not by memorization, but by the Pythagorean theorem.
2.Being able to derive these patterns myself aids in my learning because I do not have to cram in another pattern into my brain for it can remember it and if I freeze during the test and can't remember which pattern goes with what triangle I can actually take a couple of seconds and find the patterns by using the simple Pythagorean theorem and not have to fail my test.
Friday, February 21, 2014
I/D#1: Unit N Concept 7-9: Knowing all degrees,radians,and ordered pairs around the unit circle;understanding and applying ASTC to the unit circle;finding exact values of all 6 trig functions when given angle in degrees or radians;finding angles when given exact value of any 6 trig functions
INQUIRY ACTIVITY SUMMARY
Numbers #1-3 are preformed in the video.
4.This activity helps you in deriving the unit circle because the "y's" vertice(the top vertice) ends up being the ordered pair for the 30,45, 60 degree in the unit circle and those are you main repeated ordered pair of the unit circle, except the other ordered pairs are mirrored.
5. The triangle drawn in this activity are located in quadrant 1. For quadrant 2, quadrant 1 is mirrored to the left, but all of the x values are negative. For quadrant 3, quadrant 2 is mirrored down, but both the x and y values are negative. For quadrant 4, quadrant 1 is mirrored down, but the y values are negative.
In quadrant 2, the 30 degree triangle just like folded or mirrored to the left, which made the x value become negative because looking at it as in a coordinate plane, the left is in the negative. In quadrant 3 the 45 degree triangle mirrored to the left, but then mirrored down. Therefore, both the x and y values are negative because it is down and left. In quadrant 3, the 60 degree just mirrored down or if you want you can see it as if it mirrored to the left, then mirrored down, and finally mirrored to the right to make a complete circle. The x value remained positive, but the y is in the bottom right, which is negative.
In quadrant 2, the 30 degree triangle just like folded or mirrored to the left, which made the x value become negative because looking at it as in a coordinate plane, the left is in the negative. In quadrant 3 the 45 degree triangle mirrored to the left, but then mirrored down. Therefore, both the x and y values are negative because it is down and left. In quadrant 3, the 60 degree just mirrored down or if you want you can see it as if it mirrored to the left, then mirrored down, and finally mirrored to the right to make a complete circle. The x value remained positive, but the y is in the bottom right, which is negative.
INQUIRY ACTIVITY REFLECTION
1. The coolest thing I learned from this activity was the unit circle is formed by 30,45,and 60 right triangles and the unit circle's ordered pairs are all the vertices of those triangles.
2.This activity will help me in this unit because first I will not be mandated to remember all of the ordered pairs and if I decided to just remember the ordered pairs and I forget the ordered pairs of the unit I could just see the triangles and find their vertices and those will be my ordered pairs.
3.Something I never realized before about special right triangles and the unit circle is that the triangles vertices make up the unit circle and before I never applied special right triangles to anything I would just do the soh cah toa with special right triangles and now the special right triangles are applied to the unit circle.
Thursday, February 6, 2014
RWA#1: Unit M Concept 5: Graphing ellipses given equation and identifying all parts(center,focus,major and minor axis, vertices and covertices,and eccentricity)
2. An ellipse in standard form will have the equation that looks like (x-h)^2/a^2+(y-k)^2/b^2=1 or (x-h)^2/b^2+(y-k)^2/a^2. If the bigger number is under the x, then the ellipse is going to be fat. If the bigger number is under the y, then is going to be skinny. One way to remember is "y so skinny" and "you are x-tra large." An ellipse looks like a squished out/up circle. An ellipse has all of the following:center,focus,major and minor axis, vertices and covertices,and eccentricity. If you are given the general equation of an ellipse you are going to have to complete the square(it has to equal one) in order to get the equation into standard form. Once, you have it into standard form you can right away get the center. Remember that x goes if h and y goes with k and the signs will switch. Now looking at the denominator of the equation you have to see if the bigger number is under the x, then the ellipse is going to be fat and if the bigger number is under the y, then it is going to be skinny. Again looking back at the denominator of the equation, the bigger number is always "a" but you need to take the square root of that number. The other number is "b" and you also take the square root of that number. In order to find "c" you will need to plug in the two variables that you know into the formula a^2-b^2=c^2 and solve for c. To find the vertices you will have to look at your center and whether your graph is going to be fat or skinny. If your graph is fat then then you add and subtract "a"to x of the center and y will stay the same and vice versa goes if your graph will be skinny. Therefore, your major axis will be y= the y of the center(the major axis has the vertices,center, and focus)and vice versa goes if your graph will be skinny. To find the co vertices you will have to look at your center and whether your graph is going to be fat or skinny. If your graph is fat then then you add and subtract "b" to y of the center and x will stay the same and vice versa goes if your graph will be skinny. Therefore, your minor axis will be x= the x of the center and vice versa goes if your graph will be skinny. Major axis are solid lines and minor axis are dotted lines. To find the eccentricity you plug in "c" and "a" into the formula:e=c/a. In order to find the foci you have to look at your center and whether your graph is going to be fat or skinny. If your graph is fat then then you add and subtract "c"to the x of the center and y will stay the same and vice versa goes if your graph will be skinny.An ellipse has to have an eccentricity of greater than zero but less than one. Eccentricity is just "a measure of how much the conic section deviates from being circular." "As the distance between foci increases the eccentricity increases, or the reverse relationship."
To get more information on how to find the parts of an ellipse watch below.
3. A real world application of an ellipse is an extracorporeal shockwave lithotripsy, which "enables doctors to treat kidney and gall stones without open surgery"(http://www.lupdirect.com/urologicalprocedures_lithotripsy.php)
The extracorporeal shockwave lithotripsy has half of a three dimensional representation of an ellipse piece that is sitting on the patient's side. The lithotripter works because of the reflectiveness of an ellipse.
4.Works Cited
- http://www.lessonpaths.com/learn/i/unit-m-conic-sections-in-real-life/conic-sections-in-real-life
- http://www.lupdirect.com/urologicalprocedures_lithotripsy.php
- http://www.sophia.org/tutorials/unit-m-concept-5a?cid=embedplaylist
Subscribe to:
Comments (Atom)












